Cyclic Voltammetry (CV)

Posted 1/4/2016 in Uncategorized

This article is part of the AfterMath Data Organizer Electrochemistry Guide

Cyclic voltammetry involves sweeping the potential of the working electrode linearly with time at rates typically between 10 mV/s and 1000 V/s. The current is plotted as a function of potential to yield a voltammogram.

Synonyms: Cyclic linear potential sweep chronoamperometry

Detailed Description

Like most of the other electrochemical techniques offered by the AfterMath software, this experiment begins with an induction period. During the induction period, a set of initial conditions is applied to the electrochemical cell and the cell is allowed to equilibrate to these conditions. The default initial condition involves holding the working electrode potential at the Initial Potential for a brief period of time (i.e., 3 seconds).

After the induction period, the potential of the working electrode is swept linearly at the specified Sweep Rate to a Vertex Potential. Upon reaching the vertex potential, the direction of the sweep is reversed, and the sweep continues back to a Final Potential.

After the sweeping has finished, the experiment concludes with a relaxation period. The default condition during the relaxation period involves holding the working electrode potential at the final potential for an additional brief period of time (i.e., 1 seconds).

At the end of the relaxation period, the post experiment idle conditions are applied to the cell and the instrument returns to the idle state.

Current is plotted as a function of the potential applied to the working electrode, resulting in a voltammogram.

Parameter Setup

The parameters for this method are arranged on various tabs on the setup panel. The most commonly used parameters are on the Basic tab, and less commonly used parameters are on the Advanced tab. Additional tabs for Ranges and post experiment idle conditions are common to all of the electrochemical techniques supported by the AfterMath software.

Basic Tab

You can click on the “I Feel Lucky” button (located at the top of the setup) to fill in all the parameters with typical default values. You will no doubt need to change the Initial Potential, Vertex Potential, Final Potential, and Sweep Rate to values which are appropriate for the electrochemical system being studied.

Figure 1: Basic Cyclic Voltammetry Setup

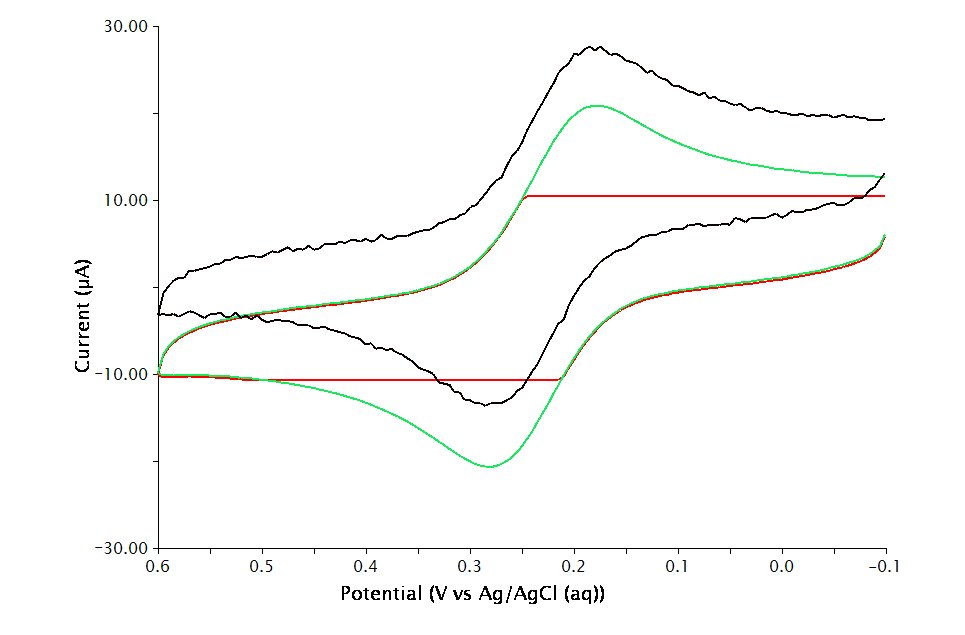
The Electrode Range on the Basic tab is used to specify the expected range of current. Consider performing cyclic voltammetry (using a $3 \; mm$ GC working electrode and sweep rate = $100 \; mV/s$ ) on a $1 \; mM$ solution of $K_3Fe(CN)_6$ in $0.1 \; M \; KCl$ . If the choice of electrode range is too small (e.g. $10 \; {\mu}A$ ), actual current may go off scale and be truncated (see Figure 2, red trace). If the electrode range is too large (e.g. $5 \; mA$ ), the voltammogram may have a noisy, choppy, or quantized appearance (see Figure 2, black trace). With the adequate electrode range (e.g. $200 \; {\mu}A$ ), the volatammogram has a desirable appearance (see Figure 2, green trace)

Some Pine potentiostats (such as the WaveNow and WaveNano portable USB potentiostats) have current autoranging capability. To take advantage of this feature, set the electrode range parameter to “Auto”. This allows the potentiostat to choose the current range “on-the-fly” while the voltammogram is being acquired.

Figure 2 : Influence of Current Range Choice on Voltammogram Quality

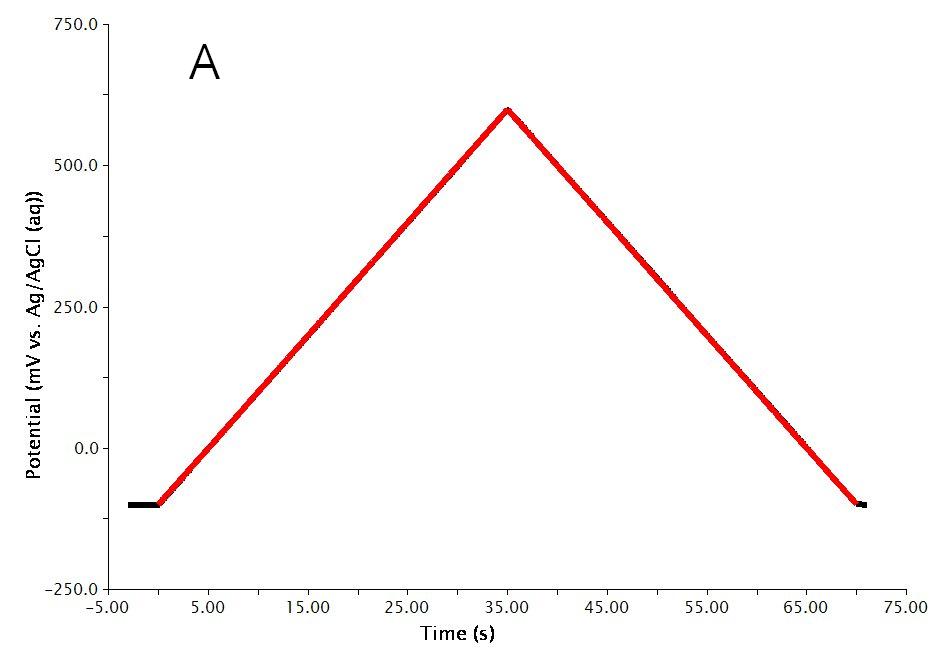
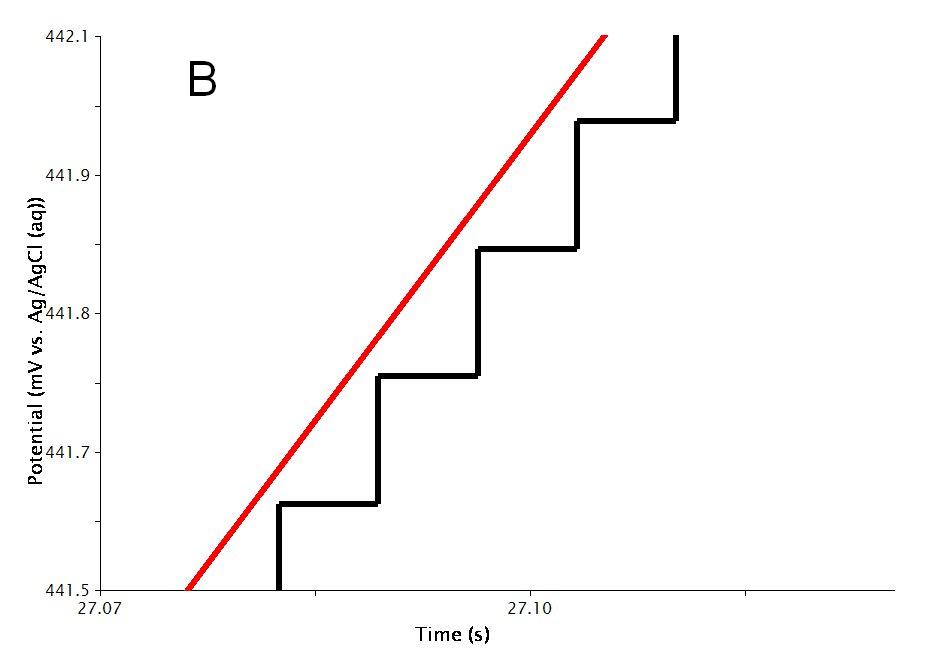
The actual waveform that is applied to the electrode is linear but not truly analog (see Figure 3A). The flat portions at the beginning and end of the waveform are the induction and relaxation periods, respectively. A typical two segment sweep starts at an initial potential, sweeps to a vertex potential, and then returns to a final potential. The sweep is not perfect; in reality is consists of many small steps (see Figure 3B, black trace) which are generated using the maximum available resolution of the potentiostat’s digital-to-analog converter (DAC).

Figure 3: Cyclic Voltammetry Waveform Details: Total waveform (A), Zoom of waveform showing steps (B)

While cyclic voltammograms are typically two segments or one cycle, it is possible to choose any number of segments for an experiment. If you choose any odd number of segments greater than two (see Figure 4), the parameters that must be entered are a little different than the two segment case. You must choose an Initial Potential, Upper Potential, Lower Potential, and Final potential. You must also choose whether the Initial direction is rising (sweep towards Upper Potential) or falling (sweep towards Lower Potential). If the Initial direction is rising, the Final potential must be different than the Lower potential. If the Initial direction is falling, the Final potential must be different than the Upper potential.

Figure 4: Three Segment Cyclic Voltammetry

If you choose any even number of segments greater than two (see Figure 5) the parameters that must be entered are the same as the three segment case. You must choose an Initial Potential, Upper Potential, Lower Potential, and Final potential. You must also choose whether the first sweep is initially rising (sweep towards Upper Potential) or falling (sweep towards Lower Potential). If the Initial direction is rising, the Final potential must be different than the Upper potential. If the Initial direction is falling, the Final potential must be different than the Lower potential.

Figure 5: Four Segment Cyclic Voltammetry

Advanced Tab

The Advanced Tab for this method (see Figure 6) allows you to change the behavior of the potentiostat during the induction period and relaxation period. By default, the potential applied to the working electrode during the induction and relaxation period will match the initial potential and final potential, respectively, as specified on the Basic Tab. You may override this default behavior, and you may also change the durations of the induction and relaxation periods if you wish.

Other important parameters on the Advanced tab are found in the Sampling Control area. This area contains two parameters, Alpha and Threshold which control when and how samples are acquired during the sweep portion of the experiment.

Figure 6: Advanced parameters for Cyclic Voltammetry

As mentioned previously, the waveform applied to the electrode (see Figure 3) is not truly linear. The actual waveform is a staircase of small potential steps. The duration of each small step is called the step period, and the step period is automatically chosen to take full advantage of the resolution of the potentiostat’s digital-to-analog converter.

The Alpha parameter controls the exact time within the step period at which the current is sampled. A alpha value of zero means the current is sampled at the start of the step period, immediately after a new potential step is applied. An alpha value of 100 means the current is sampled at the end of the step period, immediately before the next potential step is applied.

Changing alpha will have little effect on the voltammogram for a freely diffusing species in solution; however, variations in alpha can dramatically influence the results for surface bound species, especially when using older potentiostats with low DAC resolution (i.e., 12-bit).

Newer potentiostats (such as the WaveNow and WaveNano portable USB potentiostats) have 16-bit DAC resolution, so voltammograms acquired using these instruments are less influenced by the choice of alpha value. Nevertheless, researchers who use digital potentiostats to study surface-confined electrochemical systems (rather than freely diffusing species in solution) should be aware of the influence of this parameter. Further details can be found in the literature¹ and in a related article about CBP Bipotentiostat Interface Boards.

The Threshold parameter helps you to limit the amount of data retained as the voltammogram is acquired. The threshold parameter controls the interval between samples as the potential is swept from one limit to another. By default, a data point is acquired every time the sweep moves 5 millivolts. You can change the threshold from 5 millivolts to a smaller interval (if you want to acquire more data) or to a greater interval (if you want to acquire less data).

Again, consider performing cyclic voltammetry (using a $3 \; mm$ GC working electrode and sweep rate = $100 \; mV/s$ ) on a $1 \; mM$ solution of $K_3Fe(CN)_6$ in $0.1 \; M \; KCl$ . Extreme values for the threshold parameter can lead to undesirable results (see Figure 7). A smaller threshold value, typically less than or equal to 5 millivolts, produces smoother curves and larger data files (see Figure 7, red trace, interval = $1 \; mV$ ). If you set the threshold parameter to zero, then the maximum number of data points is acquired (and the size of the resulting data file will be quite large). If you set the parameter to a very large interval (e.g. $100 \; mV$ ), then the number of points acquired will be so small that the voltammogram appears sharp and jagged (see Figure 7, black trace). Intermediate intervals can also be seen in Figure 7 (gold – $10 \; mV$ , blue = $50 \; mV$ ).

Figure 7: Influence of Sampling Threshold on Voltammogram Quality

Ranges Tab

AfterMath has the ability to automatically select the appropriate ranges for voltage and current during an experiment. However, you can also choose to enter the voltage and current ranges for an experiment. Please see the separate discussions on autoranging and the Ranges Tab for more information.

Post Experiment Conditions Tab

After the Relaxation Period, the Post Experiment Conditions are applied to the cell. Typically, the cell is disconnected but you may also specify the conditions applied to the cell. Please see the separate discussion on post experiment conditions for more information.

A principle result of interest from a cyclic voltammogram is the peak current of the voltammogram. The peak current increases with the sweep rate (see Figure 8, conditions are $1 \; mM$ solution of $K_3Fe(CN)_6$ in $0.1 \; M \; KCl$ with sweep rates = $20$ , $50$ , $100$ , $250$ , and $500 \; mV/s$ for red, gold, green, blue, and black traces, respectively). The peak current is proportional to the square root of the sweep rate (see Figure 8 insert).

Figure 8: Influence of Sweep Rate on a Cyclic Voltammogram
(inset – current vs square root of sweep rate)

The peak current can be obtained by right-clicking on the trace and selecting Add Tool » Peak Height (see Figure 9). This places a peak measurement tool on the voltammogram (not always in the correct place, see Figure 10), and you can move the position of the peak tool using the control points on the tool.

Figure 9: Initial Placement of Peak Height Tool on Voltammogram

Figure 10: Right-Click to Change the Tool Properties

An important part of measuring the peak current is to use the proper baseline. In general, a small portion of the voltammogram leading up to the peak is used as the baseline. This small portion is extrapolated underneath the peak.

Right-clicking on one of the peak tool’s control points will bring up a dialog box where you can change the properties of the tool (see Figure 11). On this dialog box, there is a drop-down menu where you can choose the type of baseline. In this example, the “Free Sloped” baseline type was used. You can then manipulate the tool to draw the desired baseline and obtain an accurate peak height (see Figure 12).

Figure 11: Selection of Baseline

Figure 12: Extrapolation of Baseline for Peak Height Tool

Theory

A brief summary of the theory of cyclic voltammetry will be covered here. Randles² and Sevcik³ contributed to the development of the theory for cyclic voltammetry, however, credit for the modern treatment and notation goes to Nicholson and Shain⁴. Additionally, Bard and Faulkner⁵ provide a nice summary and description of cyclic voltammetry.

Consider a reaction, $O+e \;{\rightleftharpoons}\;R$ , with a formal potential $E^{\circ}$ . If a potential sweep is started sufficiently more positive than $E^{\circ}$ and swept negatively a non-faradaic current will initially flow. As the potential of the electrode approaches $E^{\circ}$ , $O$ begins getting reduced to $R$ creating a concentration gradient and therefore flux (mass transfer) to the surface increases. As $E$ passes $E^{\circ}$ , the concentration of $O$ at the surface of the electrode is nearly zero and mass transfer is at a maximum. The current will begin to tail off as the potential is swept to the vertex potential upon which the sweep is reversed.

The concentration of $R$ at the surface of the electrode is now very high and oxidation can proceed once $E$ begins to approach $E^{\circ}$ . An inverse of the process described above happens resulting in a similarly shaped but inverted $i-E$ curve. Ideally, the peak-to-peak separation for the reduction and oxidation waves should be $59 \: mV$ for a one-electron electrochemically reversible process. Additionally, the peak height $i_p$ in amperes $(A)$ can be described by the Randles-Sevcik equation, shown below,

$i_p=0.4463NFAC({nF{\upsilon}D}/RT)^{1/2}$

where $n$ is the number of electrons, $F$ is Faraday’s Constant $(96485 \:C/mol)$ , $A$ is the electrode area, $D$ is the diffusion coefficient, $C$ is the concentration, $R$ is the universal gas constant $(8.314 J/mol{\cdot}K)$ , $T$ is the absolute temperature $(K)$ and ${\upsilon}$ is the sweep rate. At $25^{\circ}C$ , with $A$ in $cm^{2}$ , $D$ in $cm^{2}/s$ , $C$ in $mol/cm^{3}$ and ${\upsilon}$ in $V/s$ , the above equation simplifies to

$i_p=2.69\times10^5n^{3/2}AD^{1/2}C\upsilon^{1/2}$

A good rule of approximation is $i_p$ will be appoximately $200\:{\mu}A/cm^{2}{\:}area/mM$ .

Application

Cyclic voltammetry is used extensively and the following two examples highlight its usefulness.

The first example shows that you can calculate electron transfer rate constants using cyclic voltammetry. The theory for calculating electron transfer rate constants from cyclic voltammetry was originally developed by Nicholson⁶ but the example here is from more recent work by Weber and Creager⁷. Ferrocene was irreversibly adsorbed in the form of a self-assembled monolayer to a Au surface using a long-chain alkylthiol. Varying the sweep rates gave increasingly larger $E_{peak} - E^0$ values ( $\eta$ , overpotential), with $E_{peak}$ being the oxidation or reduction peak and $E^0$ being the formal potential for ferrocene in this system. Rate constants were then calculated from these overpotentials. The authors went on to compare the experimentally obtained voltammograms to theoretically calculated voltammograms and from these were comparisons were also able to obtain reorganization energies.

In the second example, Duah-Williams and Hawkridge used cyclic voltammetry to study the temperature dependence of the kinetics of $CN^-$ dissociation from the cyanomyoglobin complex ( $Mb(II)CN$ )⁸. By comparing the experimentally obtained cyclic voltammograms to theoretically calculated cyclic voltammograms, they were able to estimate the dissociation rate, $k_f$ , by varying the sweep rate and monitoring the disappearance of an oxidation wave, associated with $Mb(II)CN$ as $CN^-$ dissociated. This is a good example of how cyclic voltammetry can give information, such as a dissociation constant, that has been traditionally been measured by NMR.